Product: Abaqus/CFD
Time accuracy, laminar flow, surface output, time-history data, spatial and temporal accuracy.
Two-dimensional laminar flow over a cylinder is a well-documented fluid dynamics problem, which makes it suitable for verification and validation purposes. This problem is characterized by boundary layer separation due to adverse pressure gradients induced by the cylinder geometry. The flow is characterized by a Reynolds number (
) defined with a free-stream velocity, V, and cylinder diameter, D, where 
and 
are the dynamic viscosity and density of the fluid, respectively. At sufficiently high Reynolds number, 
, the flow becomes unsteady and is characterized by vortices shed from either side of the cylinder in an alternating manner. The resulting downstream wake pattern is known as a Karman vortex street. The frequency at which the vortices are shed is characterized by a nondimensional parameter known as the Strouhal number (
), where f is the vortex shedding frequency. Experimental studies have demonstrated that the Strouhal number is Reynolds number dependent (Roshko, 1954), indicating that despite the simple geometry, the flow is far from being simple.
This problem was selected as an Abaqus/CFD verification problem because of the simple geometry, the unsteady dynamics, and the availability of experimental and numerical results for comparison. Specifically, we consider the case of 
flow as our benchmark problem for which the Strouhal number, 
, is obtained from the experimental data using the correlation formula given by Roshko (1954). The objective of the study is to reproduce the unsteady structure of the flow and to measure the convergence rate of Abaqus/CFD.
The model consists of a two-dimensional cylinder in a rectangular domain, as shown in Figure 3.3.1–1. The inflow boundary is located 8D upstream of the cylinder axis, the outflow boundary surface is located 25D downstream of the cylinder axis, and the top and bottom surfaces are each located 8D away from the cylinder axis. The thickness of the cylinder is 1.5D in the spanwise direction.
Mesh:
The domain topology (see Figure 3.3.1–2) is partitioned into two regions: the cylinder region, which is the box bounded by 
and 
with the origin located at the center of the cylinder (circle); and the far field and wake region that cover the complement of the domain.
In this study four meshes with varying element sizes, summarized in Table 3.3.1–1, are employed. Mesh refinement is conducted primarily on the cylinder region and in the narrow wake region behind the cylinder (see Figure 3.3.1–3).
Table 3.3.1–1 Mesh description.
| Mesh | Number of elements | h/D |
|---|---|---|
| 1 | 1740 | 0.7689 |
| 2 | 3900 | 0.5875 |
| 3 | 7800 | 0.4663 |
| 4 | 14900 | 0.3758 |
denotes the volume of a given element i, and 
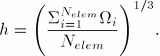
is the number of elements in the mesh.Boundary conditions:
The boundary conditions applied to the model are shown schematically in Figure 3.3.1–4.
At the inflow surface (
) the fluid velocity is specified in component form as (
) = (V, 0, 0). At the top and bottom surfaces (
) a tow-tank velocity condition is specified as () = (V, 0, 0). At the outflow surface (
) an outflow boundary condition (traction free) is specified by setting the pressure p = 0 (the gradients of velocities are automatically set to zero for this boundary). On the cylinder surface (
) a no-slip/no-penetration boundary condition is enforced, given by () = (0, 0, 0). Finally, the two-dimensional nature of the problem is enforced by prescribing the out-of-plane velocity, 
, to be zero everywhere on the domain surface and by using only one element through the thickness along the cylinder axis. Initial conditions:
The velocity, V, is set to zero everywhere in the flow domain. Velocity initial conditions that satisfy the solvability conditions for the incompressible Navier-Stokes equations are obtained by inserting the boundary conditions in the prescribed initial velocity field, followed by a projection to a divergence-free subspace. This mass adjustment to the velocity initial conditions is necessary to guarantee that the flow problem is well posed.
Problem set up:
The fluid density, cylinder diameter, inflow velocity, and dynamic viscosity values are specified as = 1 kg /m3, D = 1 m, V = 1 m/s, and = 0.01 kg/ms, respectively. These values yield a Reynolds number of 100. A transient flow simulation is performed with the total simulated time specified as t = 1000 s for Mesh 1 and t = 600 s for Meshes 2–4. The solver options are set to the defaults with the exception of the pressure Poisson equation (PPE) solver tolerance, which is set to 10–8 (default 10–5).
This verification study is intended to assess the time accuracy of Abaqus/CFD for a 
flow where a Hopf bifurcation results in steady, periodic vortex shedding. Experimental data and well-established numerical calculations are used as benchmark solutions to compare with the results obtained here.
The time at which vortex shedding first develops depends on the mesh quality. For all meshes used in this study, a periodic vortex shedding system is fully established around 200 s, after which numerical calculations were conducted for a period of 800 s for Mesh 1 and 400 s for Meshes 2–4 to collect time-history data for the drag coefficient, 
, and velocity, 
. These data were collected at a distance of approximately 4D downstream of the cylinder.
The time history signals were analyzed using a numerical discrete Fast Fourier Transform (FFT) to extract the dominant frequency. For this moderate-
case, there is only one dominant frequency (corresponding to the Hopf bifurcation) that can be computed directly by counting the number of zero-crossings during the time sample. Both approaches yielded effectively the same results.
Figure 3.3.1–5 indicates the four locations, 
= (4, 8, 12, 16), marked in red, where the time history of y-velocity is collected for Mesh 4. Figure 3.3.1–6 shows the velocity time history at these four locations. The time history results indicate that the amplitude of the velocity fluctuations decreases as the sampling point moves farther away from the cylinder, caused in part by viscous dissipation and in part by upscaling/coalescence of the vortical structures. However, the frequency of oscillations remains constant at all locations. Figure 3.3.1–7 shows a 50-second span of the time history data for Mesh 4 and reveals the periodic variation in drag induced by the vortex shedding. Here, it is important to point out that the frequency of a vortex-shedding cycle is equal to one-half of the frequency of the drag signal. Results for the rest of the meshes exhibit the same behavior; thus, these results are not presented here.
is the Fourier transform of the drag coefficient and 
is its complex conjugate. The drag coefficient is defined as
is the drag force (integrated force in the direction of the flow) and b is the spanwise dimension of the cylinder. Figure 3.3.1–8 shows the spectrum versus Strouhal number computed for Mesh 4, which indicates that the dominant frequency is located at 
.Following the same procedure, the Strouhal numbers computed for all meshes employed in this study are summarized in Table 3.3.1–2. The results for the finest mesh, Mesh 4, are in good agreement with the experimental results, St = 0.167 (1.8% difference) and with the results of Engelman and Jamnia (1990) (–1.73% difference), which are summarized in Table 3.3.1–3 for comparison.
Table 3.3.1–3 Calculated Strouhal number from benchmark calculations (Engelman and Jamnia, 1990).
| Mesh |  |  |
|---|---|---|
| Coarse | 0.296 | 0.172 |
| Medium | 0.264 | 0.172 |
| Fine | 0.266 | 0.173 |
To further assess the spatial accuracy of the code, the mean drag coefficient (obtained by averaging the time signal) is used with a Richardson extrapolation to estimate the convergence rate. Figure 3.3.1–9 shows the convergence of the drag coefficient as a function of the mesh metric (h) based on the volume, and the results are summarized in Table 3.3.1–4. In addition, the benchmark solution taken from Engelman and Jamnia (1990) is summarized in Table 3.3.1–5.
Table 3.3.1–4 Calculated mean drag coefficient.
| Mesh | h/D |  |
|---|---|---|
| 1 | 0.7689 | 1.4626 |
| 2 | 0.5875 | 1.4465 |
| 3 | 0.4663 | 1.4370 |
| 4 | 0.3758 | 1.4319 |
Table 3.3.1–5 Calculated mean drag coefficient from benchmark calculations (Engelman and Jamnia, 1990).
| Mesh | h/D | |
|---|---|---|
| Coarse | 0.7791 | 1.405 |
| Medium | 0.6930 | 1.410 |
| Fine | 0.6128 | 1.411 |
The rate of spatial convergence of Abaqus/CFD can be estimated using the results of the four meshes implemented. Following ASME V&V 20-2009, the error in the numerical solution can be computed as
, is obtained as
, such that 
= 1.4207. This value is close to the experimental data of 
reported by Wieselsberger (1922). Here, the experimental data are used to calculate the error in the calculations. Figure 3.3.1–10 presents the absolute value of the error for the drag prediction. Results indicate that error decays with a rate consistent with the second-order spatial accuracy of Abaqus/CFD, illustrated by the line with slope 
that is plotted on top of the results. Following ASME V&V 20-2009, the observed convergence between the two calculations can be approximated as
and 
, with 
. Table 3.3.1–6 shows the convergence rate computed using the equation above. The unsteady incompressible flow over a cylinder was successfully computed using Abaqus/CFD. The vortex shedding frequencies computed were found to be in good agreement with the experimental data and previous numerical calculations. Furthermore, the estimated convergence rate for the Abaqus/CFD drag coefficient was measured and was found to be in close agreement with the theoretical second-order accuracy of the code.
Mesh 1: coarse mesh with 1740 elements.
Mesh 2: mesh with 3900 elements.
Mesh 3: mesh with 7800 elements.
Mesh 4: fine mesh with 14900 elements.
ASME V&V 20-2009, “Standard for Verification and Validation in Computational Fluid Dynamics and Heat Transfer,” American Society of Mechanical Engineers.
Engelman, M. S., and M. A. Jamnia, “Transient Flow Past a Circular Cylinder: A Benchmark Solution,” International Journal for Numerical Methods in Fluids, vol. 11, pp. 985–1000 (1990).
Roshko, A., “On the Development of Turbulent Wakes from Vortex Streets,” National Advisory Committee for Aeronautics, Washington, D. C., Report 1191, 1954.
Wieselsberger, C., “New Data on the Laws of Fluid Resistance,” NACA-TN-84, 1922.
